Fill in the blanks.
(i) The centre of a circle lies in ____________ of the circle. (exterior/ interior)
(ii) A point whose distance from the centre of a circle is greater than its radius lies in __________ of the circle. (exterior/ interior)
(iii) The longest chord of a circle is a _____________ of the circle.
(iv) An arc is a ___________ when its ends are the ends of a diameter.
(v) Segment of a circle is the region between an arc and _____________ of the circle.
(vi) A circle divides the plane, on which it lies, in _____________ parts.
Ans:
(i) interior .
(ii) exterior .
(iii) diameter
(iv) semicircle
(v) chord
(vi) 3 (three) .
2. Write True or False. Give reasons for your solutions.
(i) Line segment joining the centre to any point on the circle is a radius of the circle.
(ii) A circle has only a finite number of equal chords.
(iii) If a circle is divided into three equal arcs, each is a major arc.
(iv) A chord of a circle, which is twice as long as its radius, is the diameter of the circle.
(v) Sector is the region between the chord and its corresponding arc.
(vi) A circle is a plane figure.
Ans:
(i) True.
Any line segment drawn from the centre of the circle to any point on its circumference is called the radius of the circle and will always have the same length.
(ii) False.
A circle can have an infinite number of equal chords since there are infinite points on the circumference to draw such chords.
(iii) False.
When a circle is divided into three equal arcs, none of them can be classified as major arcs because major arcs are greater than half the circle, and these arcs are all equal.
(iv) True.
A chord with a length twice the radius always passes through the centre of the circle and is called the diameter.
(v) False.
A sector is the region enclosed by two radii and the arc between them, not by a chord.
(vi) True.
A circle is a 2D geometric shape that lies on a plane.
3. Recall that two circles are congruent if they have the same radii. Prove that equal chords of congruent circles subtend equal angles at their centres.
Ans:
To recall, a circle is a collection of points whose every point is equidistant from its centre. So, two circles can be congruent only when the distance of every point of both circles is equal from the centre.
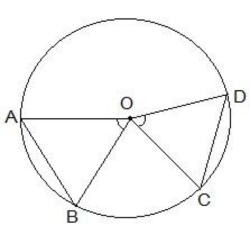
For the second part of the question, it is given that AB = CD, i.e., two equal chords.
Now, it is to be proven that angle AOB is equal to angle COD.
Proof:
Consider the triangles ΔAOB and ΔCOD.
OA = OC and OB = OD (Since they are the radii of the circle.)
AB = CD (As given in the question.)
So, by SSS congruency, ΔAOB ≅ ΔCOD
∴ By CPCT, we have,
∠AOB = ∠COD (Hence, proved).
4. Prove that if chords of congruent circles subtend equal angles at their centres, then the chords are equal.
Ans:
Consider the following diagram.

Here, it is given that ∠AOB = ∠COD, i.e., they are equal angles.
Now, we will have to prove that the line segments AB and CD are equal, i.e., AB = CD.
Proof:
In triangles AOB and COD,
∠AOB = ∠COD (As given in the question.)
OA = OC and OB = OD (These are the radii of the circle.)
So, by SAS congruency, ΔAOB ≅ ΔCOD
∴ By the rule of CPCT, we have,
AB = CD (Hence, proved.)
